



The discovery by Lee, Osheroff, and Richardson of superfluidity in liquid helium-3 completed the triad of discoveries that collectively constitute the major achievement of low temperature physics in this century. The phenomenon in helium-3 resembles and yet differs remarkably from its other two manifestations in superconductors and in the more common isotope, liquid helium-4.

Robert Richardson, David M. Lee, and Douglas Osheroff.
The impact of the discovery on subsequent physics has been immense. It has transformed the direction of theoretical and experimental research in low temperature physics, stimulating advances in our understanding of the hydrodynamics of intricately ordered systems, the microscopic theory of electrons in metals, and the range of phenomena accessible to nuclear magnetic resonance probes. Some have even seen in it suggestive implications for phenomena in astrophysics.
Two and a half decades later their achievement shines as the last great conquest on the low-temperature frontier. Surely some day it will be surpassed, but as subsequent explorations at the lowest achievable temperatures fell back from that pinnacle, theirs has now acquired the same quality as a piece of science as the human presence on the moon has acquired as a piece of technology: a standard from a golden age, against which future achievements are to be judged.
Here is the context for the discovery. The Bardeen-Cooper-Schrieffer theory of superconductivity, now so firm a piece of physics that the possibility of a new mechanism for superconductivity at higher temperatures has been hailed as revolutionary, had been in existence for only 15 years in 1972. While by 1972 the theory was no longer controversial, one of its most dramatic predictions had not been confirmed: that the same kind of self-organization responsible for the superconductivity of metallic electrons, should result in a new kind of superfluidity in the rare isotope helium-3, similar only in name to the superfluidity that had been known for decades to exist in the more common helium-4. An international quest for this holy grail had become embarassingly unsuccessful, as each new advance in the technology of reaching lower temperatures failed to reveal the expected superfluid behavior. By the 1970's the search had proceed to temperatures a thousand times lower than the temperature at which helium-4 becomes superfluid, repeatedly defying the predictions of theorists.
Lee, Richardson, and Osheroff found the signature of the transition not in the hydrodynamics of helium-3, but in its magnetic behavior. Their discovery was the culmination of a program in low temperature physics, started single-handedly by Lee a decade earlier, which had already achieved worldwide recognition. They and their competitors immediately recognized the significance of what they had seen, and work in all the great low temperature laboratories of the world was diverted to the further study of both the magnetic and the hydrodynamic properties of what still, 25 years later, is without question the world's most remarkable form of condensed matter. Superfluid helium-3, which the original discovery already revealed to come unexpectedly in two varieties (called the A-phase and the B-phase), combines in one material the quantum phase coherence of a conventional superconductor with the orientational ordering of a liquid crystal, as well as exhibiting a variety of spectacular magnetic, acoustic, and hydrodynamic properties that simply do not exist in any other known materials. The original discoverers continued to play a major role in the subsequent exploration and elucidation of these many diverse properties.
Nobel prizes have been awarded for work in low temperature physics in 1913 (Kamerlingh Onnes), 1962 (Landau), 1972 (Bardeen, Cooper, and Schrieffer), 1973 (Josephson), 1978 (Kapitsa), and 1987 (Bednorz and Muller). They have all been for work on superfluidity or superconductivity. The discovery of Lee, Osheroff, and Richardson ranks with the loftiest peaks in this tradition. It has afforded the pairing theory of Bardeen, Cooper, and Schrieffer a new test of an entirely different kind, it has provided new and spectacular examples of Josephson's phase coherence, it has afforded entirely novel tests of the two-fluid hydrodynamics invented by Landau to account for the very different superfluidity of helium-4, it has enlarged the arena of phenomena from which to search for a theoretical understanding of the discoveries of Bednorz and Muller, and it has provided the first example of a neutral syhstem, consisting of whole atoms, displaying the same strange behavior that Kamerlingh Onnes was so startled to find, more than 80 years ago, in the electronic behavior of metals.
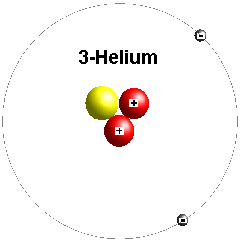
Helium-3 is stable isotope of helium with a nucleus comprised of 2 protons and 1 neutron. Some molecules are much more difficult to cool into super fluids than others are and helium-3 is one of them. It has to be cooled to 2 mK (0.002 K) before it exhibits any super fluid properties. That's a thousand times colder than Helium-4's lambda point, the temperature at which helium-4 changes from liquid to super fluid.
.
The helium-3 nucleus is a fermion rather than a boson. That means it contains an odd number of spin ½ particles. Fermions are often associated with matter and include elementary particles such as electrons and quarks (quarks are what neutrons and protons are made of). Composite particles such as protons and neutrons, with half-integer spins are also called fermions. Unlike bosons, only one fermion can occupy a particular quantum state at a time.
Fermions such as helium-3 follow Fermi-Dirac statistics and should not actually be condensable in the lowest energy state. For this reason superfluidity should not be possible in helium-3 which, like helium-4, can be liquidised at a temperature of some degrees above absolute zero. But fermions can in fact be condensed, but in a more complicated manner. This was proposed in the BCS theory which is based on the fact that electrons are fermions (they consist of one particle only, an odd number) and therefore follow Fermi-Dirac statistics just as helium-3 atoms do. But electrons in greatly cooled metals can combine in twos to form what are termed Cooper pairs and then behave as bosons. These pairs can undergo Bose-Einstein condensation to form a Bose-Einstein condensate. It was expected that the fermions in liquid helium-3 should be capable of forming boson pairs and that superfluidity should be obtainable in very cold samples of the isotope helium-3.
That superfluid 3He is the most sophisticated condensed matter system for which we can claim a quantitative understanding. It's not just a superfluid, it's an anisotropic superfluid. In an ordinary, old fashioned superconductor, he noted, "we generally believed the Cooper pairs were formed in the s state and had no interesting internal structure. The structure is more or less fixed by the thermodynamics.
There's no way you can change it. But 3He is quite different. The Cooper pairs have an internal structure, and the structure is different in the A and B phases. In 3He, both the orientation and the internal coordinates must all behave in the same way and this gives rise to spectacular effects. If I have two helium atoms and they're about to form a Cooper pair, and they're going into the A phase, they have to decide whether they're going to have their spins oriented parallel or perpendicular to that angular momentum. The energy difference between those two configurations is really tiny, of the order of a nanodegree—way below millidegrees. So, normally we would just forget about it. However, because the pairs have to be Bose condensed, they have to decide once and for all whether their spins will be parallel or perpendicular to the orbital angular momentum. So the energy in question is now multiplied by a factor of 1023. That was really fascinating. You have this tiny little thing, which is then ampli fied so spectacularly.
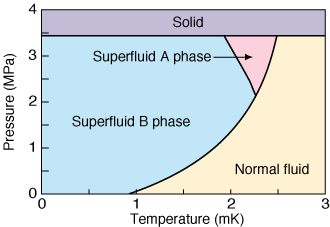
Phase Diagram.
An example of such defects are quantized vortices. The dynamics of these objects are probed using non-invasive NMR (Nuclear Magnetic Resonance) and mechanical oscillators. We study dynamic processes such as vortex motion, onset and transition to turbulence, turbulent front propagation, dissipation of turbulence, and the electromagnetic resonances of the 3He superfluid order parameter.
Most collective physical systems freeze and become immobile at zero temperature. Thus, there exist few systems where hydrodynamics can be experimentally studied in the zero-temperature limit. Most notable among these are the helium superfluids which remain in liquid state down to zero temperature and may support dissipationless superflow at sufficiently low flow velocities.
A superfluid is phenomenologically a composition of a normal component and a superfluid component. When a superfluid is brought into rotation, the non-classical behavior of the superfluid component can lead to the creation of rectilinear vortices, where the flow as a whole mimics classical solid body rotation. The main focus of our group in the past few years has been studying the dynamics of these vortices, especially both laminar and turbulent dissipation of superfluid motion.
Cell with vortices.
Another line of research is connected to Bose-Einstein condensation of quasiparticles, which has become a popular topic of studies in the recent years. In 3He suitable quasiparticles are spin waves, or magnons, which are collective bosonic excitations existing in magnetically ordered systems. In superfluid 3He it is possible to use nuclear magnetic resonance techniques to create spin states, where the magnetization in a macroscopic volume precesses around the applied magnetic field with a common phase.
Studied a Bose-Einstein condensation of magnons, which is created inside a magnetic trap. The height of the trap is controlled by externally applied magnetic field and the radial width of the trap can be modified by the angular velocity of the container. The NMR signal from the condensate can be used, e.g., to investigate the vortex dynamics at ultralow temperatures.
Already been able to solve some unsettled questions concerning trapped magnon condensates in 3He-B, in particular by controlling the trapping potential provided by the rotation of the sample. We have also studied the relaxation properties of these condensates.

Magnon condensate.
A Majorana fermion is a more than 70-years old mathematical construction, which describes a spin-1/2 particle for which the creation and annihilation operators coincide. Thus one can say that the particle is its own antiparticle. It has been realised that in the so called topological matter, such as the superfluid phases of 3He, these fermions can appear as bound states on either the container wall or in vortex cores.
One of the goals of our ongoing research is to use the NMR techniques, in which we are experts on the world-wide level, to search for both the surface-bound and core-bound Majorana states using magnon condensates. The signal of the exotic fermions should be seen among other things as a power-law type of behavior of the specific heat, opposed to the exponential dependence for the excitations in the bulk superfluid.
In future, we plan to extend the use of liquid 3He as a model system of topological matter by probing other massless fermions, the so-called Weyl and flat-band fermions in 3He-A.
REFERENCES
Cornell University. Available in: http://www.lassp.cornell.edu/Nobel/science.html. Access in: 20/10/2018.
Aalto University. Available in: http://ltl.tkk.fi/research/theory/he3.html. Access in: 20/10/2018.
Aalto University. Available in:http://ltl.tkk.fi/wiki/LT/Rota_Group. Access in: 20/10/2018.
Wikipedia. Available in: https://en.wikipedia.org/wiki/Helium-3. Access in: 20/10/2018.
Super Fluids. Available in: https://superfluidsiiti.weebly.com/helium-3.html. Access in: 20/10/2018.

Default timespace