



In 1922, German physicists Otto Stern and Walther Gerlach hypothesized that electrons behaved as tiny magnetic bars, each with a north and south pole. To test this theory, they fired a bundle of silver atoms between the poles of a permanent magnet with a stronger north pole than the south pole.
According to classical physics, the orientation of a dipole in an external magnetic field must determine the direction in which the beam is deflected. Since a magnet bar may have a range of orientations relative to the external magnetic field, they would expect to see atoms being diverted by different amounts to a given spread distribution. Instead, Stern and Gerlach observed that the atoms were clearly divided between the north and south poles.
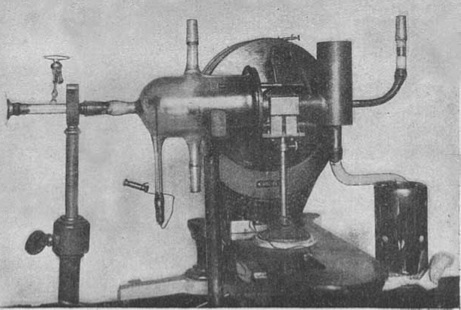
Photo of the apparatus used by Stern and Gerlach showing the key components.

The experimental scheme.

What was observed in relation to the classical prediction.
The Stern–Gerlach experiment demonstrated that the spatial orientation of angular momentum is quantized, and thus an atomic-scale system having intrinsically quantum properties. In the original experiment, silver atoms were sent through a spatially varying magnetic field, which deflected them before they struck a detector screen, such as a glass slide. Particles with non-zero magnetic moment are deflected, due to the magnetic field gradient, from a straight path. The screen reveals discrete points of accumulation, rather than a continuous distribution owing to their quantized spin. Historically, this experiment was decisive in convincing physicists of the reality of angular-momentum quantization in all atomic-scale systems.
Sometimes we represent the spin of the electron by drawing the electrons as arrows pointing up, ↑, or down, ↓. A consequence of the electron spin is that a maximum of 2 electrons can occupy any orbital, and the 2 electrons occupying the same orbital have opposite spins. This is also called Pauli exclusion principle. The spin also influences the magnetic properties that a material presents, such as paramagnetism and diamegnetism represented below using the orbital fill model.

.
Quantum number of electronic spin magnetic, ms:
An electron on an atom has the expected magnetic properties for a rotated charged particle. The "spin" of the electron must be represented by a fourth quantum number, ms.
REFERENCES
SEARS; ZEMANSKY; YOUNG; FREEDMAN. Física IV. Ótica e Física Moderna. 12ª Ed. Pearson, São Paulo. p. 274-279.
Wikipedia. Available in: https://en.wikipedia.org/wiki/Stern%E2%80%93Gerlach_experiment. Access in: 17/09/2018.

Default timespace